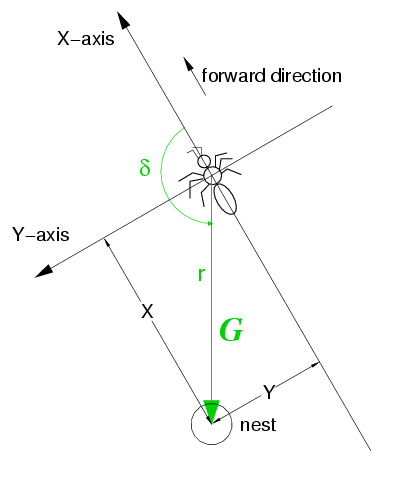
Desert arthropods (e.g. the ant Cataglyphis fortis, the beetle Parastizopus armaticeps, or the woodlouse Hemilepistus reaumuri) return to their nest on a straight path even after a long and tortuous foraging excursion. This ability is based on an internal "path integrator", i.e. the integration of walking speed and angular variation along the walking route during foraging.This mechanism provides the animal with the distance and direction of the nest from its actual position at any time, the so called home vector. Playing out this home vector allows the arthropod to return to the nest on a straight path. Nevertheless, after beelining back the arthropod only rarely hits spot on the entrance of its nest. In this case, it starts a systematic search behaviour in the vicinity of its supposed nest, while extending the search radius more and more.Together with Wolfgang Alt and Martin Rost, I have developed a model that can perform the process of mathematically correct path integration. This model uses a combination of two coupled differential equations to calculate the arthropod's distance from the nest in cartesian coordinates. The model is egocentric, i.e. it has no fixed point of origin but the point of origin is the moving arthropod itself (see figure on the left). This is biologically plausible as the physiological sensing and locomotion apparatus of an arthropod is bound to its body. Therefore, it is naturally related to its two symmetry axes, the posterior-anterior axis and the perpendicular right-left axis. Thus, when identifying these symmetry axes with the X and Y axis of a cartesian coordinate frame (X,Y) and taking the arthropod's body centre as the origin (0,0), this constitutes a proper planar moving coordinate frame for representing the relative position of any object in the planar neighbourhood of the arthropod, e.g. its nest. The figure above shows the theoretical scheme of egocentric path integration by means of cartesian coordinates X,Y, specifying the position of the nest relative to the arthropod's body axes and determing the vector G = (X,Y), here with X < 0 and Y > 0.
Given the arthropod's forward speed v and angular turning rate omega, one yields the following model equations for a precise update of the home vector (X,Y) during motion:
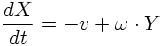
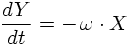
Our two-dimensional differential equation system is remarkably simple: it is linear in the two variable quantities X and Y, and it just uses the two speed input parameters as additive or multiplicative terms, v representing the rate of shift of the X coordinate backwards, and omega the rate of rotating the (X,Y) frame clockwise.
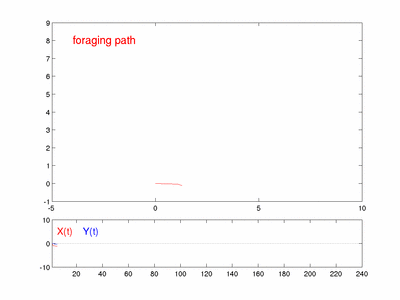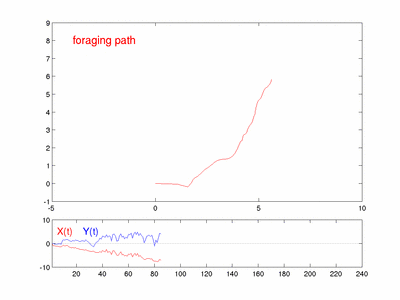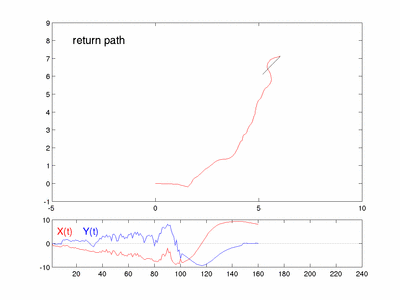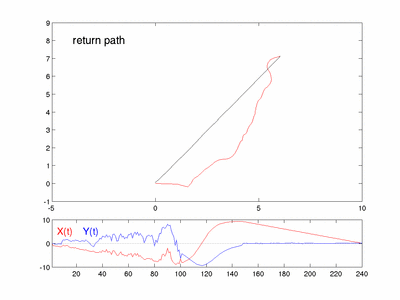
The figure displays a model simulation of a natural outbound path and the successful return path back to the nest due to precise path integration, according to our model. Top: Plot of the actual path in cartesian (x,y)-coordinates. The foraging (outbound) path is shown in red, the home run in black. Bottom: The values of X and Y over time as computed following the two differential equations (see above) provide sufficient information for the animal to return to the nest on a straight path.
For more details regarding the figures and our egocentric path integration model, please see our paper in the Journal of Theoretical Biology 2006.Recently, Robert Vickerstaff and I have started to model the systematic search behaviour of desert ants. This behaviour enables a forager to find the nest quickly even if the path integrator has failed to provide the exact position of the nest.
Project collaborators: Prof Dr Wolfgang Alt (Universität Bonn, Germany), Dr Robert Vickerstaff (University of Canterbury, New Zealand)
Path Integration and Search Strategies in Desert Ants, Cataglyphis fortis
This projecgt aims at gaining a better understanding of the intriguing navigational abilities of desert ants. Like many central-place foraging species (see above) these animals return to their nest on a fairly straight path, even when the foraging run has been very tortuous. I conducted several experiments in the Tunisian desert in close cooperation with Rüdiger Wehner (Universität Zürich). These experiments focused on the accuracy and precision of the path integrator, its interaction with visual markers such as landmarks, and the systematic search strategies these ants use as final backup mechanism to find the nest.
Project collaborator: Prof Dr Rüdiger Wehner (Universität Zürich, Switzerland)
Accuracy and Precision of the Path Integrator
Desert ants use path integration not only to return to the nest, a tiny hole in the desert ground (see left image), as efficiently as possible but also to return to valuable food sources. Therefore, it is possible to establish feeders and to train the animals to forage to and fro between their nest and this feeder (see picture in the middle, where a colour-marked Cataglyphis fortis leaves the feeding sites with a biscuit crumb). Marking the ants individually enables an experimenter to compare the successive foraging trips of individual ants. Also, the training allows for testing the accuracy of the path integrator of groups of ants that differ in their foraging routines, e.g. after they have been trained to feeders that were at different positions. By painting white lines on the desert ground (see right image) I was able to record the ants' trajectories on graph paper with a reduced scale. Paths were digitised and transformed into cartesian coordinates for analysis.
Using these techniques, we could prove the long held assumption that the path integrator accumulates errors during foraging: the longer the foraging trip has been, the larger the overall error in the path integrator. Also the experiments revealed that repeated training does not improve the path integrator in desert ants but even after an ant had visited the same feeder many times, the accuracy of its outbound and inbound runs did not improve remarkably. Apparently, a high accuracy from the beginning is ecologically more sensible than fine-tuning the system during consecutive runs. In their natural environment, food sources may vanish quickly anyway.
Interaction of Path Integrator and Landmark Information
Path integration is not the only means of navigation desert ants have at their disposal. However, due to their habitat which consists mainly of bare desert ground without any vegetation, most of the time they have to rely exclusively on their path integrator. But, whenever available, they make use of visual markers. I was the first to show how the path integrator and landmark information interact during foraging excursions. As in my other experiments, I painted white lines on the desert ground in order to record the ants' paths and trained them to artificial feeders. Some groups of ants were trained on the open ground, others had parts of their outbound and inbound runs flanked by black cylinder landmarks (see image on the right). The results of those experiments showed that landmarks have a remarkable impact on the foraging motivation of desert ant foragers and also that if the information provided by the landmarks and the path integrator are set into conflict, the ants tend to trust the landmark information more than the information supplied by the path integrator.
Systematic Search in Desert Ants
If an ant fails to find the nest after following the home vector - which is provided by the path integrator - it starts a systematic search for the nest.
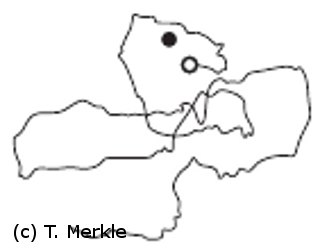During this search C. fortis performs loops of increasing radius around the supposed nest position. It regularly returns to a region that is close to the starting point of the systematic search (open circle in left figure), i.e. the nest position as calculated by the path integrator, and then changes the direction in which it heads off next until it eventually finds the correct nest position (filled circle).I compared the search patterns of groups of ants that differed regarding their foraging excursions and showed that the ants adapt their systematic search patterns to the uncertainty of the path integrator, i.e. the longer the foraging excursion - and the larger the error accumulated in the path integrator - the wider the search loops. In other words, the ant accounts for this uncertainty by adjusting their search patterns accordingly. An additional experiment revealed that the searching behaviour is also influenced by the particular experience of the respective ant such as having foraged on familiar terrain. In summary, these experiments brought evidence that the systematic search in Cataglyphis is not a fixed routine but a very flexible and adaptive behaviour.
© Dr. Tobias Merkle
Disclaimer